Unveiling Current Flow: Drift vs. Diffusion in Semiconductors

Demonstrative Video

Variation of Semiconductor parameters with temperature

Variation of semiconductor parameters with temperature:
- Intrinsic concentration \(\left(n_i\right)\)
- mobility \(\left(\mu\right)\)
- conductivity \(\left(\sigma\right)\)
- Energy gap \(\left(E_G = E_C-E_V\right)\)
\[\begin{aligned} \text{electron conc.} ~ n & = N_c \cdot \mathrm{e}^{{-(E_C-E_F)/kT}} \\ \text{Hole conc.}~p & = N_V \cdot \mathrm{e}^{-(E_F-E_V)/kT}\\ n_i^2 & = n\cdot p = N_CN_V \cdot \mathrm{e}^{-(E_C-E_V)/kT} \\ & = N_CN_V \cdot \mathrm{e}^{-E_G/kT} \end{aligned}\]
Note: \(n_i\) is independent of Fermi level but depends on \(T\) and \(E_G\)

\(n_i\) is ver sensitive to \(T\) and is given by:

\[\begin{aligned} A_o&= \text{a constant, independent of temperature}\\ T&= \text{temperature}~ \left(\right. \left.{ }^{\circ} \mathrm{K}\right)\\ E_{G O}&= \text{forbidden energy gap at}~ { }^{\circ} \mathrm{K} (\text{in eV}) \\ k&= \text{Boltzmann constant}~ \left( \mathrm{eV} /{ }^{\circ} \mathrm{K}\right) \end{aligned}\]

\[n_i^2 = A_oT^3\mathrm{e}^{-E_{Go}/kT}\]

\(T\) Effect on Extrinsic semiconductor:
- \(T\uparrow~\Rightarrow~n_i^2\uparrow ~\Rightarrow\) effect charge density
- N-type: \(n\) does not change appreciably but \(p \uparrow\)
- P-type: \(p\) constant, \(n\uparrow\)

Mobility (\(\mu\))

\[m = \left.\begin{cases} \text{Silicon} \Rightarrow & \text{electrons} (2.5), ~\text{holes}(2.7) \\ \text{Germanium} \Rightarrow & \text{electrons} (1.66), ~\text{holes}(2.33) \end{cases} \right\}\]

\[\propto T^{-m} \quad T~\text{varies from 100 to 400}^\circ K\]

Intrinsic semiconductor: \(T\uparrow \Rightarrow \mu \downarrow\)
\(\mu\) is a function of Electric field intensity (E [V/m])

\[\mu = \left.\begin{cases} E < 10^{3} & \text{constant} \\ 10^{3} < E < 10^{4} & \propto E^{-0.5} \\ \text{higher field} & \propto 1/E \end{cases} \right\}\]

Conductivity(\(\sigma\))
- depends on number of electron-hole pairs and mobility
- \(T\uparrow\) number of e-p pairs \(\uparrow\) and \(\mu~\downarrow\)
- number of e-p pairs \(>~\mu\)
- At T\(^\circ{K}\) where \(\alpha\) is temperature coefficient
- Intrinsic semiconductor: T \(\uparrow~\Rightarrow~\sigma~\uparrow\)
- Extrinsic semiconductor: T \(\uparrow~\Rightarrow~\sigma~\downarrow\) as the number of majority carriers is constant and \(\mu~\downarrow\)

\[\sigma = \sigma_0\left[1+\alpha(T-T_0)\right]\]

Energy Gap

\[E_G(T) = E_{G0} - \beta \cdot T \qquad T~\uparrow \Rightarrow E_G(T)~\downarrow\]

\[\begin{aligned} \beta & = \text{constant} = \begin{cases} \text{Si} & 3.6 \times 10^{-4} \\ \text{Ge} & 2.23 \times 10^{-4} \end{cases}\\ E_{G0} & = \text{Energy gap at}~ 0^{\circ}\mathrm{K} \quad = \begin{cases} \text{Si} & = 1.21~ \mathrm{eV}\\ \text{Ge} & = 0.785~ \mathrm{eV} \end{cases} \end{aligned}\]

Drift and Diffusion Currents

Flow of charge (current) through a semiconductor or PN junction diode has two components:
1. Drift current
2. Diffusion current
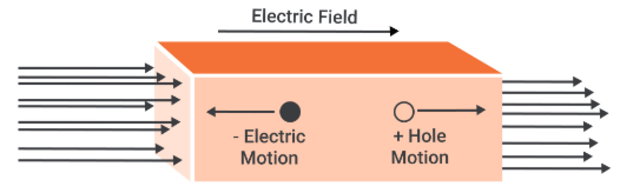
Drift current:
- Drift current arises from the movement of carriers in response to an applied electric field.
- Positive carriers (holes) move in the same direction as the electric field
- Negative carriers (electrons) move in the opposite direction.
- The net motion of charged particles generates a drift current that is in the same direction as the applied electric field.

\[\text{Drift current density,}~J ~\text{A/cm\textsuperscript{2}} = \begin{cases} J_n = qn\mu_n E ~\Rightarrow \text{due to free electrons} \\ J_p = qp\mu_p E ~\Rightarrow \text{due to holes} \\ \end{cases}\]

\[\begin{aligned} n &= \text{number of free electrons per cubic centimetre} \\ p & = \text{number of holes per cubic centimetre} \\ \mu_n & = \text{mobility of electrons in} ~\mathrm{cm}^2 / \mathrm{V}-s \\ \mu_p & = \text{mobility of holes in} ~\mathrm{cm}^2 / \mathrm{V}-\mathrm{s} \\ E & = \text{applied electric field intensity in}~ \mathrm{V} / \mathrm{cm} \\ q & = \text{charge of an electron} =1.6 \times 10^{-19} ~\text{coulomb} \\ \end{aligned}\]

Diffusion current :
- Electric current can flow even in the absence of applied voltage provided a concentration gradient exists
- When the number of either electrons or holes is greater in one region than that of other region
- Charge carrier move from higher to lower concentration of same type of charge carrier
- Movement of charge carrier resulting in a current called diffusion current

\[\text{Diffusion current density}~J~\text{A/cm\textsuperscript{2}} = \begin{cases} J_p = -qD_p\dfrac{dp}{dx} \Rightarrow \text{hole}\\ \\ J_n = -qD_n\dfrac{dn}{dx} \Rightarrow \text{electron} \end{cases}\]

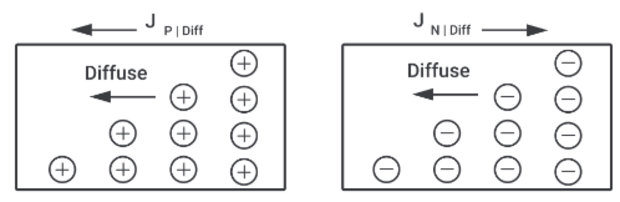
hole density \(p(x)\) decreases with increasing \(x\), hence negative sign
\(J_p\) is positive in \(+x\) direction
\(dn/dx\) and \(dp/dx\) are concentration gradients
\(D_n\) and \(D_p\) are diffusion coefficients in cm²/s
\[= \begin{cases} J_p=q p \mu_p E-q D_p \dfrac{\mathrm{d} p}{\mathrm{~d} x} \\ \\ J_n=q n \mu_n E-q D_n \dfrac{\mathrm{d} n}{\mathrm{~d} x} \end{cases}\]
Total current :

Einstein Relationship :
- Relationship between mobility and diffusion coefficient of a particular type of charge carrier in the same semiconductor
- Higher the charge carrier mobility, greater will be its tendency to diffuse
- Used to determine \(D_{p,n}\) by experimentally measuring \(\mu_{p,n}\)

\[\frac{D_p}{\mu_p}=\dfrac{D_n}{\mu_n}=\dfrac{k T}{q}=V_T\]