Essential Formulas for DC Machines

Notations

\[ \begin{aligned} P & = \text{Number of Poles} \\ \Phi & = \text{Flux/pole} \\ N & = \text{Speed of Armature, RPM}\\ Z & = \text{Number of armature conductors} \\ A & = \text{Number of parallel paths} \\ \omega & = \text{Angular speed (rad/sec)} \\ V & = \text{Terminal Voltage (V)} \\ I & = \text{Terminal Current (A)} \\ E & = \text{Induced EMF (V)} \\ I_a & = \text{Armature Current (A)} \\ I_{sh} & = \text{Shunt Field Current}~\mathrm{A} \\ I_{se} & = \text{Series Field Current}~\mathrm{A} \\ R_a & = \text{Armature Resistance}~\Omega \\ R_{se} & = \text{Series Field Resistance}~\Omega \\ R_{sh} & = \text{Shunt Field Resistance}~\Omega \\ T & = \text{Torque, Nm} \\ \end{aligned} \]

EMF Equation of DC Machine

\[ E = \dfrac{\Phi P N}{60} \times \dfrac{Z}{A} \]

Types of DC Machines

Shunt-Wound: Field windings are in parallel with the armature.
Series-Wound: Field windings are in series with the armature.
Compound-Wound: Combination of series and shunt windings.

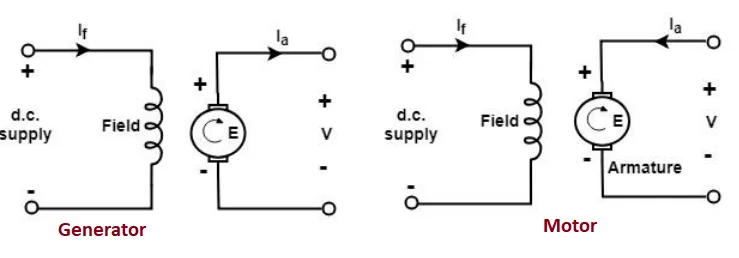
Separately Excited DC Machine

Self-Excited DC Machines

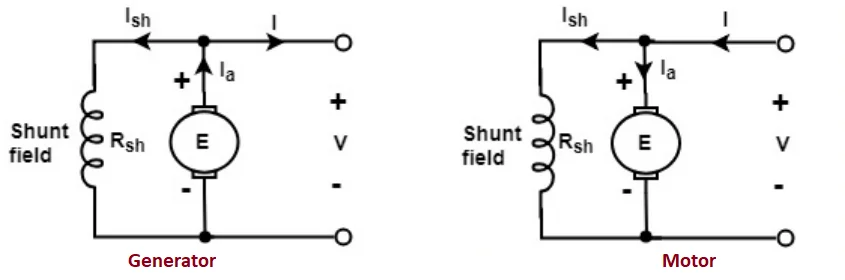
Shunt DC Machine

Generator	Motor
\[\begin{aligned} E & =V+I_{a}R_{a}+V_{brush}\\ I_{a} & =I_{sh}+I\\ I_{sh} & = \dfrac{V}{R_{sh}} \end{aligned}\]	\[\begin{aligned} V & =E+I_{a}R_{a}+V_{brush}\\ I & =I_{sh}+I_{a}\\ I_{sh} & = \dfrac{V}{R_{sh}} \end{aligned}\]

Series Wound DC Machine

Generator	Motor
\[\begin{aligned} E & = V + I_a \left(R_a+R_{se}\right) + V_{brush} \\ I_a & = I \end{aligned}\]	\[\begin{aligned} V & = E + I_a \left(R_a+R_{se}\right) + V_{brush} \\ I_a & = I \end{aligned}\]

Short-Shunt DC Machine

Generator	Motor
\[\begin{aligned} E & = V+I_aR_a+IR_{se}+V_{brush} \\ I_a & = I+I_{sh}\\ I_{sh} & = \dfrac{V+I\cdot R_{se}}{R_{sh}} \end{aligned}\]	\[\begin{aligned} V & = E+I_aR_a+IR_{se}+V_{brush} \\ I & = I_a+I_{sh}\\ I_{sh} & = \dfrac{V-I\cdot R_{se}}{R_{sh}} \end{aligned}\]

Long-Shunt DC Machine

Generator	Motor
\[\begin{aligned} E & = V + I_a \cdot (R_a + R_{se}) +V_{brush} \\ I_a & = I+I_{sh} \\ I_{sh} & = \dfrac{V}{R_{sh}} \end{aligned}\]	\[\begin{aligned} V & = E + I_a (R_a + R_{se}) +V_{brush} \\ I & = I_a+I_{sh} \\ I_{sh} & = \dfrac{V}{R_{sh}} \end{aligned}\]

Construction Concepts

Pole Pitch

\[\text{Pole Pitch} = \frac{Z}{P}\]

\[\text{Pole Pitch (slots)} = \frac{\text{Total number of slots}}{P}\]

is the distance between the centers of two adjacent poles in terms of armature conductors: The

Commutator Pitch

The Commutator Pitch is the distance between the two commutator segments to which the ends of a coil are connected:

Lap Winding: \(Y_c = 1\)
Wave Winding: \(Y_c = \frac{2p \pm 1}{2}\)

Coil Span (Coil Pitch)

The Coil Span is the distance between the two sides of a coil in terms of armature slots:

\[\text{Coil Span (slots)} = \text{Pole Pitch (slots)}\]
: For
For Short-Pitch Winding: The span is less than the pole pitch.

Back and Front Pitch

\[Y_b = \frac{Z}{P}\]
Back Pitch (\(Y_b\))
Front Pitch (\(Y_f\)) is the distance between the beginning of one coil and the beginning of the next coil connected to the same commutator segment.

Electrical and Mechanical Degrees

\[\theta_e = \frac{P}{2} \times \theta_m\]

) is given by: ) and mechanical degrees (The relationship between electrical degrees (

Armature Reaction in DC Machines

Demagnetising and Cross-Magnetising Ampere-Turns per Pole

\[AT_{\text{dem}} = ZI \times \dfrac{\theta_m}{360^{\circ}}\]

\[AT_{\text{cross}} = ZI \left( \dfrac{1}{2P}-\dfrac{\theta_m}{360^{\circ}} \right)\]

The demagnetising ampere-turns per pole:

Compensating Winding

\[AT_{\text{comp}} = \frac{I_a Z}{2P}\]

The ampere-turns required for the compensating winding to neutralize the cross-magnetising effect:

Power Equations

Electrical Power Input

\[P_{\text{in}} = V I_a\]

Mechanical Power Output

\[P_{\text{out}} = T \omega = T \cdot \frac{2 \pi N}{60}\]

Where:

\(\omega\) = Angular speed (rad/s)

Power Developed in Armature

\[P_a = E I_a\]

Torque Equation for DC Motor

\[T = \frac{P Z \Phi I_a}{2 \pi A}\]

Where:

\(T\) = Torque (Nm)
\(I_a\) = Armature current (A)

\[T \propto \Phi I_a\]

Simplified formula:

Losses in DC Machine

Copper Losses:
- Armature copper loss: \(P_{\text{cu}} = I_a^2 R_a\)
- Field copper loss (Shunt): \(P_{\text{f}} = \frac{V^2}{R_f}\)
- Field copper loss (Series): \(P_{\text{f}} = I_a^2 R_f\)
Iron (Core) Losses:
- Hysteresis Loss: \(P_{\text{hyst}} \propto B^{1.6} f V\)
- Eddy Current Loss: \(P_{\text{eddy}} \propto B^2 f^2 t^2 V\)
Mechanical Losses: Friction and windage losses.

Efficiency of DC Machines

\[\eta = \frac{P_{\text{out}}}{P_{\text{in}}} = \frac{P_{\text{out}}}{P_{\text{out}} + \text{losses}}\]

Generator Efficiency: \(\eta = \frac{V I}{E I_a}\)
Motor Efficiency: \(\eta = \frac{T \omega}{V I_a}\)

Condition for Maximum Efficiency

\[P_{\text{cu}} = P_{\text{iron}} + P_{\text{mechanical}}\]

Maximum efficiency occurs when variable losses equal constant losses: