Demonstrative Video
Problem-1
Find the current \(I\) using Superposition theorem?
Solution-1
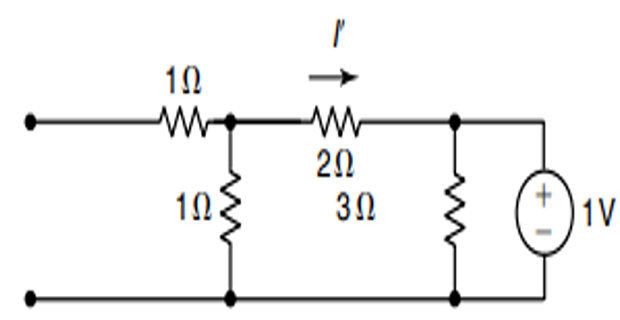
When voltage source acting alone \(I^{'} = -1/3\) A
When current source is acting alone
\[I^{''} = 1\times \dfrac{1}{1+2}=1/3~\mathrm{A}\]- \[I=(I^{'}+I^{''}) = -1/3+1/3=0\]By Superposition:
Problem-2
Use superposition theorem to determine current \(I\) ?
Solution-2
When 10-V voltage source is acting alone
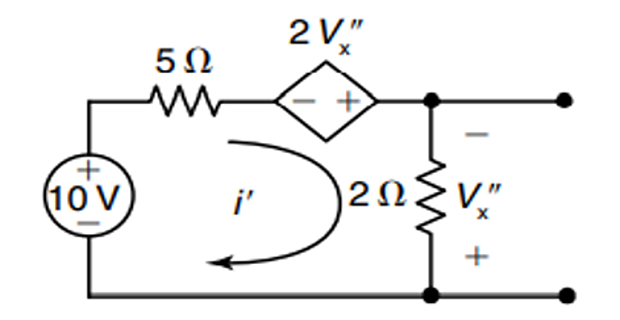\[\begin{aligned} \text{KVL} \Rightarrow 5i^{'}-2v^{'}_x+2i^{'}& = 10; \quad v^{'}_x = -2i^{'} \\ \Rightarrow 7i^{'}+4i^{'} & =10 \Rightarrow i^{'} = 10/11~\mathrm{A} \end{aligned}\]
When 2-A current source is acting alone
\[\begin{aligned} &\text{KCL} \Rightarrow 2 = i_x + i^{''} = -\dfrac{v^{'}_x}{2}+i^{''} \\ &\text{KVL} \Rightarrow 5i^{''} + 3v^{''}_x = 0\\ & \quad \Rightarrow i^{''} = -\dfrac{3}{5}v^{''}_x \end{aligned}\]
\[\begin{gathered}
2=-\frac{v_{x}^{\prime \prime}}{2}-\frac{3}{5} v_{x}^{\prime
\prime} \Rightarrow v_{x}^{\prime \prime}=-\frac{20}{11} \\
\therefore i^{\prime \prime}=-\frac{3}{5}
\times\left(-\frac{20}{11}\right)=\frac{12}{11} \mathrm{~A} \\
I=\left(i^{\prime}-i^{\prime
\prime}\right)=\left(\frac{10}{11}-\frac{12}{11}\right)=-\frac{2}{11}
\mathrm{~A}
\end{gathered}\]
Problem-3
Find \(i_0\) and \(i\) using Superposition ?
Solution-3
- \[\begin{aligned} i^{'}_0 & = i^{'} \\ \text{KVL} \Rightarrow & 6i^{'}+2i^{'}=6\\ \Rightarrow & i^{'} = i^{'}_0=0.75~\mathrm{A} \end{aligned}\]
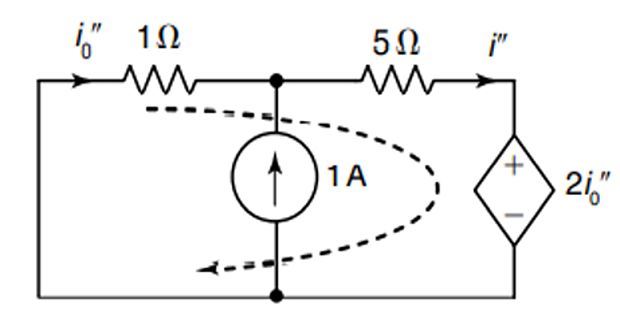
When 1-A current source is acting alone
\[\begin{aligned} \text{KCL} \Rightarrow 1&=i^{\prime \prime}-i_{0}^{\prime \prime} \Rightarrow i^{\prime \prime}=1+i_{0}^{\prime \prime} \\ \text{KVL in supermesh} \Rightarrow & 1 \times i_{0}^{\prime \prime}+5 i^{\prime \prime}+2 i_{0}^{\prime \prime}=0\\ & 3 i_{0}^{\prime \prime}+5 i^{\prime \prime}=0\\ & 3 i_{0}^{\prime \prime}+5\left(1+i_{0}^{\prime \prime}\right)=0\\ & i_{0}^{\prime \prime}=-\frac{5}{4}=-1.25 \mathrm{~A}\\ \therefore ~ & i^{\prime \prime}=1-1.25=-0.25 \mathrm{~A} \end{aligned}\]By superposition:
\[\left.\begin{array}{l} i=\left(i^{\prime}+i^{\prime \prime}\right)=(0.75-0.25)=0.5 \mathrm{~A} \\ i_{0}=\left(i_{0}^{\prime}+i_{0}^{\prime \prime}\right)=(0.75-1.25)=-0.5 \mathrm{~A} \end{array}\right\}\]
Problem-4
Find \(V_{AB}\) using Superposition theorem ?
Solution-4
- \[\begin{aligned} I^{\prime} & = 2/12 = 1/6~\mathrm{A} \\ V^{\prime}_{AB} & = I^{\prime} \times 6 = 1~\mathrm{V} \end{aligned}\]When 2-V source acting alone
When 4-V source acting alone
\[\begin{aligned} I^{''} & = 4/12 = 1/3~\mathrm{A} \\ V^{''}_{AB} & = I^{''} \times 6 = -2~\mathrm{V} \end{aligned}\]When 2-A source acting alone, convert into equivalent voltage source
\[\begin{aligned} I^{'''} & = 8/12 = 2/3~\mathrm{A} \\ V^{'''}_{AB} & = - I^{'''} \times 6 = -4~\mathrm{V} \end{aligned}\]- \[V_{A B}=V_{A B}^{\prime}+V_{A B}^{\prime \prime}+V_{A B}^{\prime \prime \prime}=(1-2-4)=-5 \mathrm{~V}\]By Superposition
Problem-5
Determine \(V_1\) using Superposition theorem ?
Solution-5
When 8-A source is acting alone
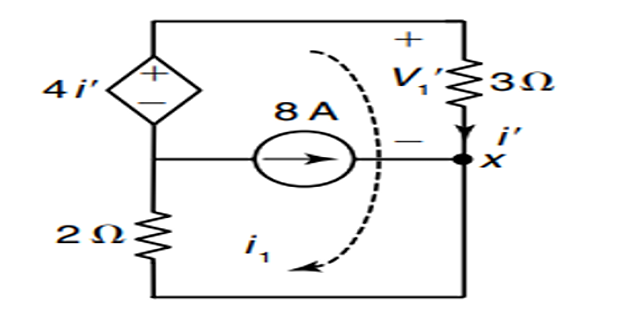
\[\begin{aligned}
\text{KVL in supermesh} \Rightarrow & 3 i^{\prime}+2 i_{1}-4
i^{\prime}=0 \Rightarrow i_{1}=\frac{1}{2} i^{\prime}\\
\text{KCL at node} x \Rightarrow &
i_{1}=\left(8+i^{\prime}\right) \Rightarrow \frac{1}{2}
i^{\prime}=8+i^{\prime} \Rightarrow i^{\prime}=-16 \mathrm{~A}
\\
\therefore ~ & V_{1}^{\prime}=3 i^{\prime}=3 \times(-16)=-48
\mathrm{~V}
\end{aligned}\]
When 2-A source is acting alone
\[\begin{aligned}
\text{KVL} \Rightarrow &
3\left(i_{2}+2\right)+2 i_{2}-4 i^{\prime \prime}=0
\Rightarrow 5 i_{2}+6-4 i^{\prime \prime}=0 \\
i^{\prime \prime}&=\left(i_{2}+2\right) \\
&\therefore 5 i_{2}+6-4\left(i_{2}+2\right)=0
\Rightarrow i_{2}=2 \mathrm{~A} \\
&\therefore i^{\prime
\prime}=\left(i_{2}+2\right)=(2+2)=4 \mathrm{~A} \\
&\therefore V_{1}^{\prime \prime}=3 i^{\prime
\prime}=3 \times 4=12 \mathrm{~V}
\end{aligned}\]
When 10-V source is acting alone
- \[\begin{aligned} &3 i^{\prime \prime \prime}-10+2 i^{\prime \prime \prime}-4 i^{\prime \prime \prime}=0 \Rightarrow i^{\prime \prime \prime}=10 \mathrm{~A} \\ \therefore & V_{1}^{\prime \prime \prime}=10 \times 3=30 \mathrm{~V} \end{aligned}\]By KVL,
- \[V_{1}=\left(V_{1}^{\prime}+V_{1}^{\prime \prime}+V_{1}^{\prime \prime \prime}\right)=(-48+12+30)=-6 \mathrm{~V}\]When all the sources are acting simultaneously, by the superposition theorem the voltage is given as
Problem-6
Find \(I\) using superposition theorem ?
Solution-6
4-V source alone
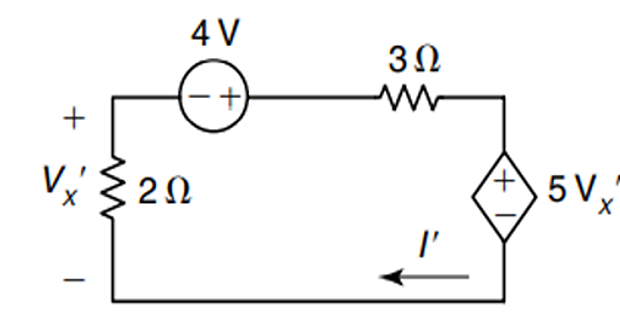
\[\begin{aligned}
\text{KVL} ~
-4+3 I^{\prime}+5 V_{x}^{\prime}-V_{x}^{\prime} &=0 \\
3 I^{\prime}+4 \times\left(-2 I^{\prime}\right) &=4 \\
\left[\because V_{x}^{\prime}=-2 I^{\prime}\right] &
\end{aligned}\]
’
2-A source alone
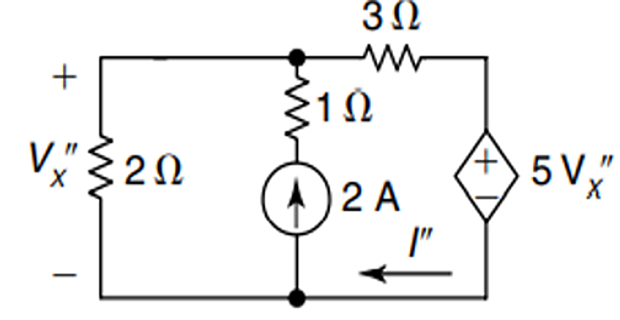
\[\begin{aligned}
\text {KCL, } & 2=\frac{V_{x}^{\prime
\prime}}{2}+\frac{V_{x}^{\prime \prime}-5 V_{x}^{\prime \prime}}{3} \\
& \Rightarrow V_{x}^{\prime \prime}=-\frac{12}{5}=-2.4
\mathrm{~V} \\
\therefore & I^{\prime \prime}=\frac{V_{x}^{\prime
\prime}-5 V_{x}^{\prime \prime}}{3}
=3.2 \mathrm{~A}
\end{aligned}\]
- \[I=\left(I^{\prime}+I^{\prime \prime}\right)=(-0.8+3.2)=2.4 \mathrm{~A}\]By superposition theorem